To be able to tell someone a distance, speed or any other magnitude, it is important to inform a unit. For example, it makes no sense to say that a person's height is two since "2" just tell us the quantity and not the scale. Now, if we inform that a person is two meters high, you can take a ruler on this unit and check the size that it corresponds to. For this reason, it is essential to inform units. And therefore, the International System of Units, whose acronym is \(IS\), which standardizes the units that are used in most countries of the world, was created. In this app, we use this system for all units.

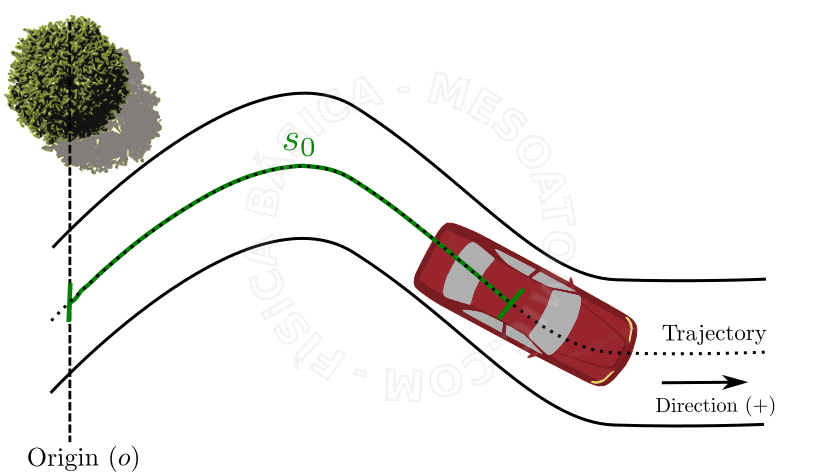
Referential

Simply put, a referential is an object (point), for which we study the changes in the position of another body.

Example 1

ANIMATION

Movement

It is the change in position over time in relation to a given reference.

Trajectory

The set of different positions that a body passes through in a given time interval. Note that the trajectory depends on the adopted reference.

Scalar Position \((s)\)

It is the distance's value, with a positive or negative sign depending on the direction adopted. This distance is measured on the trajectory, between the object and the referential's origin. In general, we begin to count time \((t=0)\) when the object is at the initial position, denoted by \(s_0\).

Variation \((\Delta)\)

For a movement description, it is important to consider the variation of some quantities, denoted by the symbol delta ( \(\Delta\) ). For example, if we leave at 6 p.m. from work and arrive at 7 p.m. at home, the variation of time \(\Delta t\) in this path will be 1h. That is, \(\Delta t = t - t_0\) where \(t_0\) is the time of the beginning of the path and \(t\) is the time of the end of the path.

Scalar Displacement\((\Delta s)\)

It is the variation (\( s - s_0\)) of the object's position, in the time interval \(\Delta t\).

Average Scalar Speed\((\bar{v})\)

During a road trip, for example, car's speed changes in different sections of the path. However, this movement can be described in a simplified manner, considering that, on average, the vehicle remains at the same speed, the average speed \(\bar{v}\). Mathematically, we have: $$\bar{v} = \frac{\Delta s}{\Delta t},$$ where \(\Delta t\) is the time spent traveling and \(\Delta s\) the distance traveled. The speed unit in the \(IS\) is meter per second, \([\bar{v}]=\frac{m}{s}\).

Example 2

Example 3

Example 4

Example 5

Example 6

Example 7

Example 8

Example 9

Average Scalar Acceleration \((\bar{a})\)

For a more accurate description of a movement, we must also consider how the speed changes in different sections. The average acceleration \(\bar{a}\) has information on how, on average, the speed changes in the path. Mathematically, this is: $$\bar{a} = \frac{\Delta v}{\Delta t},$$ where \(\Delta v\) is the speed variation in the considered path and \(\Delta t\) is the time the object took to move along the path. The \(IS\) unit of acceleration is meters per second squared, \([\bar{a}]=\frac{m}{s^2}.\)

Note that, if we segment the motion analysis using smaller and smaller time intervals, we will obtain a more accurate description of the motion as time decreases.